Making Math More Accessible Through Concrete Manipulatives and Visuals

What comes to mind when you think back to learning math in school? It would seem that most people I ask typically respond with a negative or neutral response and very few with something positive. Since many of us were taught primarily using procedures and steps, it is unlikely that too many of us could see math as anything more than rules, steps and symbols despite the fact that mathematics was created to help us better understand the world around us.

If this is so, then why aren’t we learning math first with concrete objects that we can touch and feel in order to allow students to co-construct and develop the rules, steps and symbols that represent those real world situations. By doing so, we are helping students develop the ability to visualize the mathematics they are engaging in and they will have an opportunity to see mathematics very differently to that of our generation.

Have a look at the visual below.

How many seats do you see?

I bet you see 18, right?

Here’s the fun part.

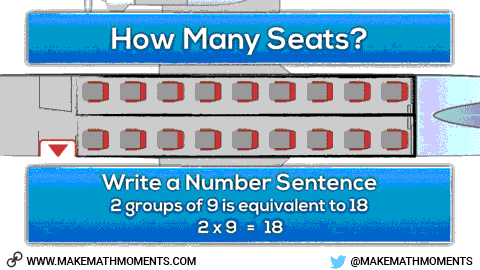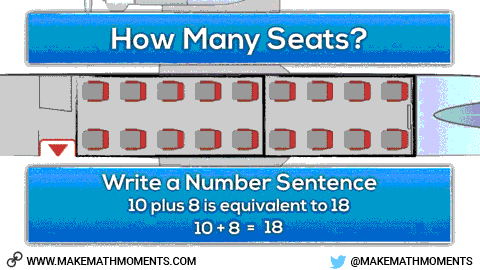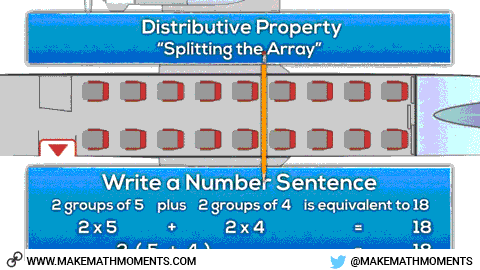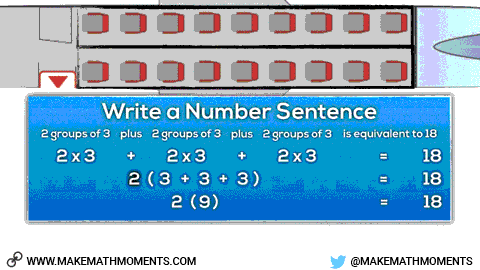
How many different ways can you write a numerical expression to represent those 18 seats. I’m going to guess that you all can come up with at least these two:

9 + 9
and
2 x 9

An assumption I’ll go with in this post is that “2 x 9” is read “2 groups of 9”. However, there are other interpretations that would match a different visual.

How many others can you come up with?

While this is a fun activity to give students practice writing expressions, the most important element here is the concrete representation (if you were using square tiles) or the visual representation (say images of the seats as we are doing here).

There are just a few of the many representations you could come up with:

By using concrete manipulatives like square tiles for this activity and allowing students to progress towards drawing visual representations when they are comfortable and able, we can give students the opportunity to build a conceptual understanding of how mathematical expressions are created and make conjectures as to what generalizations can be made about simplifying them.

While my representations are based on the assumption that a single seat represents the whole, you could also explore other scenarios such as having the entire plane represent the whole for exploring expressions with fractions, decimals and percentages.

If you’ve been trying to find a way to make math more accessible for all students in your classroom, using concrete manipulatives and visual representations is definitely a great starting point.

I plan to come back to this idea on a regular basis, so be sure to stick around for that. In the meantime, you might consider exploring some of my previous posts related to visualizing mathematics.